G.E. Hughes & M.J. Cresswell, A New Introduction to Modal Logic, London and New York: Routledge, 1996, pp. 53-56.
(2) The System S4
① S4 Lp ⊃ LLp
S4 Lp ⊃ LLp
② Translation into quantification theory

③ S4 (1) and S4 (2)
S4 (1) MMp ⊃ Mp
S4 (2) Lp ≡ LLp [R4]

④ S4 (3)-(7)
S4 (3) Mp ≡ MMp
S4 (4) MLMp ⊃ Mp
S4 (5) LMp ⊃ LMLMp
S4 (6) LMp ≡ LMLMp
S4 (7) MLp ≡ MLMLp

⑤ S4* (Lp ∧ MMq) ⊃ M (p ∧ q)
S4* (Lp ∧ MMq) ⊃ M (p ∧ q)

(3) Modalities in S4
① Modality: any unbroken sequence of zero or more monadic operators (~, L, M).
② We express the zero case by writing ‘-'.
e.g., -, ~, L, M~, LL, ~MLM
③ Standard Form: in any system containing LMI every modality can be expressed either without any negation signs at all or else with only one, and that at the beginning.
④ Iterated modality
iff it contains two or more modal operators; thus LL and ~MLM are iterated modalities, but ~ and ~L are not.
⑤ A modality is afirmative if it contains no negation signs and negative if it does contain one.
⑥ We say that two modalities, A and B, are equivalent in a given system iff the result of replacing A by B (or B by A) in any formula is always equivalent in that system to the original formula; otherwise we say that they are non-equivalent, or distinct in that system. In a system containing the rules US and Eq the modalities A and B are equivalent iff (Ap ≡ Bp) is a theorem of that system.
⑦ If A and B are equivalent in a certain system, and A contains fewer modal operators than B, then B is said to be reducible to A in that system. Clearly the formulae we have called reduction laws express the reducibility of certain modalities to others in systems of which they are theorems.
⑧ In S4 every modality is equivalent to one or other of the following or their negations:
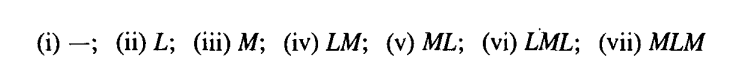
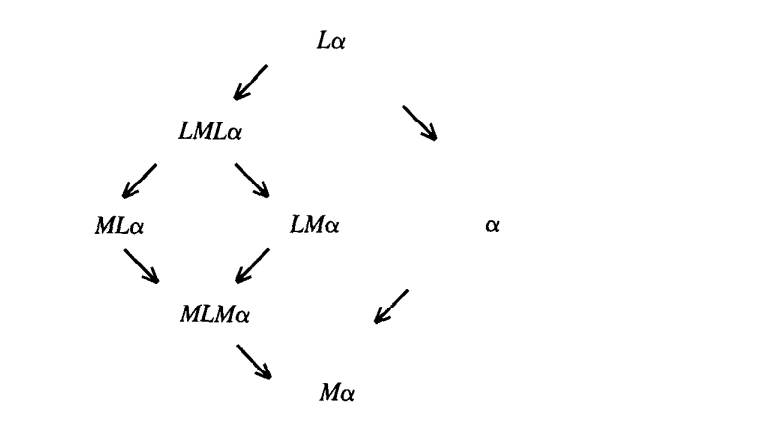
S4(2) and S4(3) entitle us to replace LL by L and MM by M; so if we add a modal operator to (ii) or (iii) we shall obtain either a modality equivalent to the original or else (iv) or (v), which are therefore the only irreducible two-operator modalities. In just the same way, if we add a modal operator to (iv) or (v), the only three-operator modalities we can obtain are (vi) and (vii). If, however, we add a modal operator to (vi) or (vii), the result is always equivalent either to the original as before, or else to (iv) or (v) by S4(6) or S4(7); hence there cannot be any irreducible modalities with four or more operators.
Clearly the negative cases can be dealt with in the same way; so what we have shown is that there are at most fourteen distinct modalities in S4. (p. 55)
The situation is strikingly different in T. The absence of any reduction laws in that system means that no matter how many modal operators a modality may contain, we can always construct a longer one which will not be equivalent to it. T therefore contains an infinite number of distinct modalities. (p. 56)
(4) Validity for S4

'Logic > Modal Logic' 카테고리의 다른 글
| Chapter 3. The Brouwerian System (0) | 2024.01.04 |
|---|---|
| Chapter 3. The System S5 (0) | 2024.01.04 |
| Chapter 3. Iterated Modalities (0) | 2024.01.03 |
| Chapter 2. The System D (0) | 2024.01.03 |
| Chapter 2. The System T (0) | 2024.01.03 |